original posted by: http://outofthisworldx.wordpress.com/2014/07/10/crop-circle-re-peace-from-a-diamond-backed-rattlesnake/
REAL UFOs Making Crop Circles Caught on Camera
Published on Apr 3, 2013
Crop circles are awesome, but how often do you catch UFOs making crop circles? Here's a 2:31 video showing the incredible phenomena of several UFOs hovering above a field in Wiltshire, England, possibly in the process of making one of the most intricate and largest crop circles discovered. I don't know how real aliens do it, but that design is pretty amazing.
Below is the original tape that contains the above clip:
REAL UFOS Filmed Making CROP CIRCLES in Wiltshire, England 07/27/10
This is the full length video starting with the UFOs hovering in the night - there is loud traffic noise - talking starts around the 3:22 mark - tape is 13:50 min long - showing only night scene with lights over field - very interesting light show! The daytime crop circle taping starts at 6:57.
.

.
Markings on a diamond-backed rattlesnake and high mathematics: who could have made a new crop picture ART at Tetbury Lane on July 8, 2014?
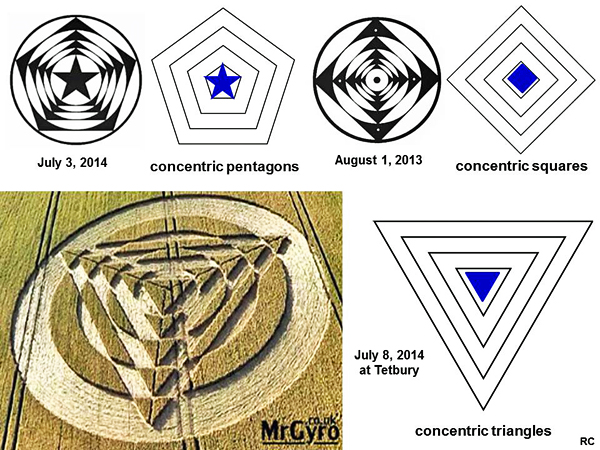
A new crop picture which appeared near Tetbury Lane in Wiltshire on July 8, 2014 resembles two other field images from 2013 or 2014:
.
.
On July 3, 2014, we saw “concentric pentagons” near the Long Man of Wilmington
.

.
One year before on August 1, 2013, we saw “concentric squares” near Stonehenge (seewww.lucypringle.co.uk).
Five days after those “concentric pentagons” appeared on July 3, 2014, we saw “concentric triangles” on July 8. Might the number series 5-4-3 be intended to suggest another paranormal event three days later on July 11?
When we study the landscape near this new crop picture at Tetbury, we can see that it was drawn in a triangular field which lies along the long body of a “landscape serpent”:
.

.
The crop picture is triangular in shape, and contains many “small arrows” which resemble the markings on a diamond-backed rattlesnake. In that landscape image, our “serpent friend” is looking down on a group of buildings near the centre of the slide above. What might this imply?
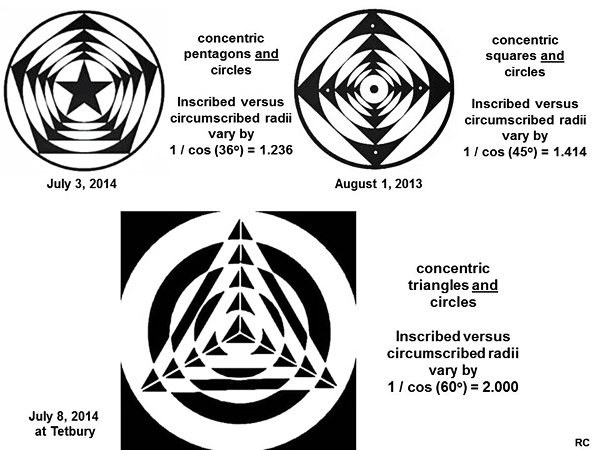
All three of these “concentric geometry” crop pictures show a combination of concentric polygons and concentric circles.
.
.
The general formula for a ratio of two radii r2 / r1, when two circles are inscribed or else circumscribed around a polygon of n equal sides, is r2 / r1 = 1 / cos (360o / 2n).
Thus for concentric circles and pentagons on July 3, 2014, the radius of each concentric circle increases going in-to-out by 1 / (cos 36o) = 1.236. Likewise for concentric circles and squares on August 1, 2013, the radius of each concentric circle increases going in-to-out by 1 / (cos 45o) = 1.414. Finally for concentric circles and triangles on July 8, 2014, the radius of each concentric circle increases going in-to-out by 1 / (cos 60o) = 2.000.
.
.
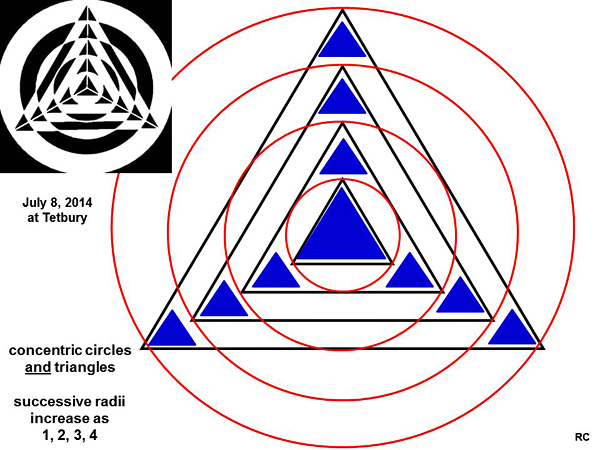
Following from that last relation, a series of four concentric triangles and circles as drawn in crops on July 8, 2014 vary in radii by r = 1, 2, 3, 4. Here “circles 1 and 2” surround the same “triangle”, while “circles 2 and 4” also surround the same “triangle”. In each case, the inscribed versus circumscribed circles vary in radii by 1 / (cos 60o) = 2.000:
As a general rule, successive “outward pointing arrows” in these three crop pictures appear to be stretched further apart, as we proceed from the case of a pentagon (n = 5) to a square (n = 4) to a triangle (n = 3). For the case of hexagon (n = 6), first studied by Archimedes in 500 BC, one would see a ratio of radii equal to 1 / (cos 30o) = 1.155, which is smaller than for the other three cases studied.
Concentric circles and triangles have been used not only to calculate “pi”. When many different concentric circles show successive areas of π, 2π. 3π, 4π and so on, then they provide a graphical demonstration of the “Wallis product” from advanced mathematics (seecommonsensequantum.blogspot.com.au or exhaustion-of-nested-squares-and-wallis):
.

.
Who could have made this spectacular new crop picture ART near Tetbury Lane on July 8, 2014?
Surely the same crop artist who made two others in 2013 or 2014 in diverse parts of England!
He represents himself as a “serpent”, and is an expert at higher mathematics and geometry.
Can we think of anyone who fits those characteristics?
.
THOU ART
PEACE
.

.
Markings on a diamond-backed rattlesnake and high mathematics: who could have made a new crop picture at Tetbury Lane on July 8, 2014?
A new crop picture which appeared near Tetbury Lane in Wiltshire on July 8, 2014 resembles two other field images from 2013 or 2014:
On July 3, 2014, we saw “concentric pentagons” near the Long Man of Wilmington
.

.
One year before on August 1, 2013, we saw “concentric squares” near Stonehenge (seewww.lucypringle.co.uk).
Five days after those “concentric pentagons” appeared on July 3, 2014, we saw “concentric triangles” on July 8. Might the number series 5-4-3 be intended to suggest another paranormal event three days later on July 11?
When we study the landscape near this new crop picture at Tetbury, we can see that it was drawn in a triangular field which lies along the long body of a “landscape serpent”:
The crop picture is triangular in shape, and contains many “small arrows” which resemble the markings on a diamond-backed rattlesnake. In that landscape image, our “serpent friend” is looking down on a group of buildings near the centre of the slide above. What might this imply?
All three of these “concentric geometry” crop pictures show a combination of concentric polygons and concentric circles.
The general formula for a ratio of two radii r2 / r1, when two circles are inscribed or else circumscribed around a polygon of n equal sides, is r2 / r1 = 1 / cos (360o / 2n).
Thus for concentric circles and pentagons on July 3, 2014, the radius of each concentric circle increases going in-to-out by 1 / (cos 36o) = 1.236. Likewise for concentric circles and squares on August 1, 2013, the radius of each concentric circle increases going in-to-out by 1 / (cos 45o) = 1.414. Finally for concentric circles and triangles on July 8, 2014, the radius of each concentric circle increases going in-to-out by 1 / (cos 60o) = 2.000.
Following from that last relation, a series of four concentric triangles and circles as drawn in crops on July 8, 2014 vary in radii by r = 1, 2, 3, 4. Here “circles 1 and 2” surround the same “triangle”, while “circles 2 and 4” also surround the same “triangle”. In each case, the inscribed versus circumscribed circles vary in radii by 1 / (cos 60o) = 2.000:
As a general rule, successive “outward pointing arrows” in these three crop pictures appear to be stretched further apart, as we proceed from the case of a pentagon (n = 5) to a square (n = 4) to a triangle (n = 3). For the case of hexagon (n = 6), first studied by Archimedes in 500 BC, one would see a ratio of radii equal to 1 / (cos 30o) = 1.155, which is smaller than for the other three cases studied.
Concentric circles and triangles have been used not only to calculate “pi”. When many different concentric circles show successive areas of π, 2π. 3π, 4π and so on, then they provide a graphical demonstration of the “Wallis product” from advanced mathematics (see commonsensequantum.blogspot.com.au or exhaustion-of-nested-squares-and-wallis):
Who could have made this spectacular new crop picture near Tetbury Lane on July 8, 2014?
Surely the same crop artist who made two others in 2013 or 2014 in diverse parts of England!
He represents himself as a “serpent”, and is an expert at higher mathematics and geometry.
Can we think of anyone who fits those characteristics?
.
PEACE |

No comments:
Post a Comment